Equipped with a world-class design engineering team, an expert technical project support team, and an advanced manufacturing facility outfitted with state-of-the-art optics fabrication, lens assembly and metrology systems, we have what it takes to provide custom optics and lens assemblies for complex optical instruments. One of our areas of expertise is in the design and manufacture of cylindrical lenses.
The following article provides an overview of cylindrical lenses, outlining how they work, the different types available, and typical applications.
How Do Cylindrical Lenses Work?
Cylinder lenses are optical components that have different radii in the x and y axes, which cause them to have a cylindrical/semi-cylindrical shape and image magnification along only one axis. This unique design allows light from a laser, or other illumination source, to be focused into a one-dimensional line instead of a point. Depending on the design of the lens, they can be used to condense or expand light in one dimension. Similar to all optical lenses, cylindrical lenses are used to manipulate light to suit the optical requirements of the intended application.
The optical properties of cylindrical lenses make them well-suited for a wide range of applications. Common use cases are:
- Creating a single laser line in laser scanning and optical metrology inspection systems
- Shaping light beams for imaging applications (e.g., 3D volumetric microscopy)
- Correcting for astigmatism in imaging systems
- Creating symmetrical output beams for laser diodes using two cylinder lenses set orthogonal to one another (e.g., circularizing an elliptical beam)
Types of Cylindrical Lenses
Cylindrical lenses are available in numerous variations to suit different optical requirements, including:
- Singlets, Doublets, Triplets. Single, double, and triple optical assemblies consist of one, two, or three lens elements, respectively
- Plano-Convex/Plano-Concave. Plano-convex lenses condense light along a single axis, while plano-concave lenses expand light along a single axis.
- Sphero-Cylindrical. Sphero-cylindrical lenses have one surface shaped like a sphere and one surface shaped like a cylinder.
- Symmetric Convex/Concave. In symmetric cylindrical lenses, both faces have the same curvature radius. The faces can be either convex or concave.
- Acylinders. Acylinder lenses combine aberration-reducing benefits of an aspheric surface with the one-dimensional focusing of a cylinder lens.
Industrial Applications of Cylindrical Lenses
Cylindrical lenses and assemblies find application in a wide range of industries. Some of the industrial sectors that regularly employ cylindrical lenses are:
- Industrial Inspection. Cylindrical lenses are used to focus or expand light in a variety of laser and light-based equipment for applications, such as laser scanning optical metrology systems, laser alignment and inspection tools, and laser additive manufacturing and laser processing equipment.
- Semiconductor Manufacturing. For semiconductor manufacturing, plano-convex cylindrical lenses are often used in conjunction with plano-concave cylindrical lenses to circularize elliptical beams. Other semiconductor industry applications include wafer inspection tools and flat panel display measurement tools.
- Entertainment. In the entertainment industry, cylindrical optical assemblies are used in digital cinema cameras to compress widescreen images so they fit on standard 35 mm film and digital/laser projectors to stretch compressed images so they can be viewed normally when projected onto a widescreen.
- Life Sciences and Medical. Cylindrical lenses are used in a variety of life science imaging and medical test instruments, such as light sheet fluorescence microscopy (LSFM) and ophthalmological systems.
Cylindrical Lens Solutions From JML Optical
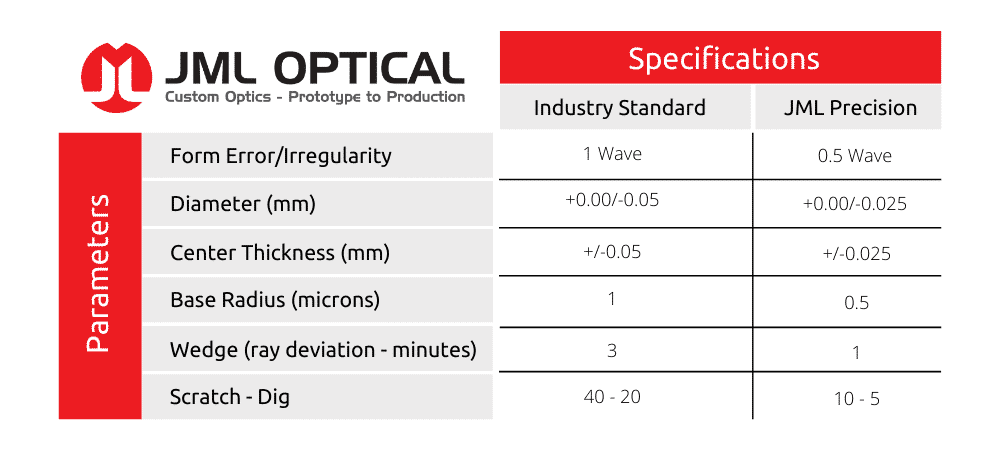
At JML Optical, we utilize advanced CNC machining and traditional X-Y axis fabrication techniques in conjunction with metrology equipment to produce precision-engineered cylindrical lenses. We accommodate customer requests for cylindrical lenses and lens assemblies with the following specifications:
- Sizes: 10mm–635 mm
- Material Types: all optical glass, fused silica, quartz
To discuss your cylindrical lens requirements with one of our experts, contact us or request a quote today.